Edinburgh Elasto-Plastic Adhesion Model (EEPA)

The Edinburgh Elasto-Plastic Adhesion Model (EEPA) contact model captures the history dependence and the key characteristic behavior of cohesive solids.

The flow behavior and handling characteristics of cohesive granular solids are strongly dependent on the prior consolidation stress experienced by the solid.
This model comprises a nonlinear hysteretic spring model to account for the elastic-plastic contact deformation and an adhesive force component that is a function of the plastic contact deformation. The EEPA offers a versatility that allows it to be used in both linear and non-linear modes (Thakur, et al. 2014). When two particles or agglomerates are pressed together, they undergo elastic and plastic deformations. It is assumed that the pull-off strength (adhesion) increases with an increase of the plastic contact area. The contact force-displacement relationship for microscopic particles are shown in the following figure. For more information, see (Jones, 2003), (Jones, 2004).
Figure 1.


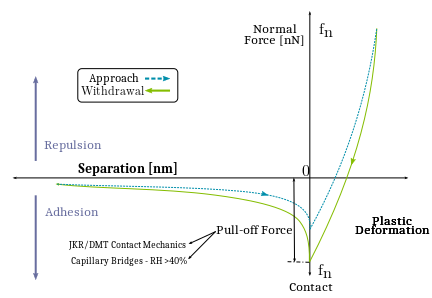
The force-separation curves from AFM is a schematic illustration of observed force-separation curves for adhesive particles, as adapted from Jones:

In addition to the base interaction parameters in EDEM (Coefficient of Restitution, Static Friction and Rolling Friction) there are six additional parameters to configure for the EEPA model.

The loading, unloading, and adhesive conditions are described by the following six parameters:
  • Constant pull-off force, f0 (N)

    Used to incorporate ever presents forces that may occur, such as van der Waals type forces or electrostatic force. The magnitude of this force will not change for the simulation duration.

  • Meso-contact Adhesion Energy, Δγ (J/m2)

    Defined as the level of adhesion that is used in the calculation of the load dependent adhesive force in the contact model, fmin.

  • Contact Plasticity Ratio, λp

    Defined as (1-k1/k2), this is the level of contact plasticity used in the model. This parameter defines the unloading/reloading stiffness k2 as a constant ratio between the initial stiffness k1 and the unloading/reloading stiffness k2. A value of zero represents elastic particle contacts, while a value of one leads to perfectly plastic particle contacts.

  • Power Value for k1 and k2 F-D relationship, n

    This is used to switch between linear and non-linear force-overlap relationships for the contact model.

  • Power value for adhesion branch, X

    Defined as the severity of the drop in adhesion force following the peak tensile force being reached. A value of unity gives a linear, more ductile separation curve, whereas a sharp drop in strength is found for values bigger than X = 2, which is similar to the separation behavior depicted in Figure 1.

  • Tangential Stiffness multiplier, ζtm

    Allows the tangential stiffness used in the model to be varied. The tangential stiffness is defined as a ratio of the Virgin Loading Stiffness, k1 – a multiplier of one gives a tangential stiffness equal in magnitude to the loading stiffness.

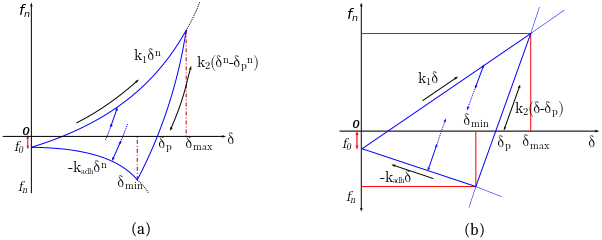
The following figure demonstrates the linear and non-linear contact force-overlap relationship - schematic of the non-linear (a) and linear (b) contact force–displacement function (Thakur, et al. 2014).
Figure 2.


Model Versatility

  1. The non-linearity of the loading and unloading branches is controlled by the parameter n. When n=1, there is linear behavior (Fig.2 (b)), and the model replicates a linear spring, whereas when n=1.5, the model replicates the Hertzian Spring model.
  2. If the level of contact plasticity, λp, is set to zero then the magnitude of the virgin loading stiffness k1 and the unloading/reloading stiffness k2 are equal and the model reverts to being an elastic model.
  3. The tangential stiffness multiplier, ζtm, defines the ratio of the tangential to normal stiffness and varies depending on the contact model being used. For the case of linear elastic particle contact a value of 1 equates the normal and tangential stiffness. The ratio of tangential to normal stiffness for real elastic materials lies in range of 2/3 to 1, and depends on Poisson’s ratio (Mindlin 1949). You should consult the relevant literature for exact values of ζtm. Also, the presented values may not necessarily apply for cohesive, plastic contacts.
These parameters are retained as constants for the duration of the simulation. You can change the parameters at any time once the simulation is stopped and then restart the simulation.