Okumura-Hata Propagation Model

The Okumura-Hata propagation model is a simple empirical model with short computation time.

With this model, only the transmitter and receiver height are processed. The terrain profile between transmitter and receiver is not considered. If, for example, a hill is located between transmitter and receiver, its shadowing effect is not taken into account.

Click Project > Edit Project Parameter and click the Computation tab.

Parameters

Because of the calibration with measurement data, the Okumura-Hata-model is restricted to the following ranges for the different parameters:
  • frequency f (150...1500 MHz)
  • distance between transmitter and receiver d (1...20 km)
  • antenna height of the transmitter h t x (30...200 m)
  • antenna height of the receiver h r x (1...10 m)

As the height of the transmitter and the receiver is measured relative to the ground, an effective T x antenna height ( h e f f ) is determined to account for the topographical impact. The transmitter antenna height above ground Hb, used in both the formulas of the Okumura-Hata propagation model and in the formulas of the Extended Hata model, is equal to h t x if h e f f ≤ h t x , and is equal to h e f f if h e f f > h t x . This improves the accuracy of the prediction.

Figure 1. Effective antenna height for the Okumura-Hata propagation model.


Settings

ProMan offers two variations for the computation:
  • Homogenous environment: For the full prediction area, a homogeneous environment is considered without the settings in the morpho / clutter database.
  • Individual environment: As defined in morpho / clutter database.
    Figure 2. The Parameters of Okumura Hata dialog.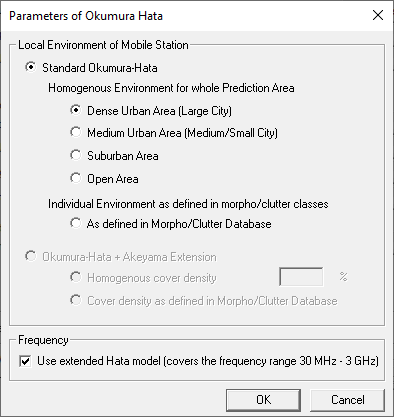


Computation

The following equations show the computation of the basic path loss (in dB) with the model of Hata-Okumura.

L=69.55+26.16log(f)−13.82log( H b )−a( H m )+( 44.9−6.55log( H b ) )log(d)

Where,
  • L = path loss (dB)
  • f = frequency (MHz)
  • Hb = transmitter antenna height above ground (m)
  • Hm = receiver antenna height above ground (m)
  • d = distance between transmitter and receiver (km)
  • a(Hm) = antenna height correction factor
defined as follows:

For medium urban area (medium, small city):

a( H m )=( 1.1log(f)−0.7 ) H m −( 1.56log(f)−0.8 )

For dense urban area (large city):

a( H m )=8.29 [ log(1.54 H m ) ] 2 −1.1     if   f≤200
a( H m )=3.2 [ log(11.75 H m ) ] 2 −4.97   if   f≥400

For the suburban or open environment, a correction factor is taken into account as in the following formulas:
L suburban =L{ urban }−2 [ log( f 28 ) ] 2 −5.4
L open =L{ urban }−4.78 [ log(f) ] 2 +18.33[ log(f) ]−40.94
with the following model restrictions:
  • f: 150 MHz to 1500 MHz
  • Hb: 30 m to 200 m
  • Hm: 1 m to 10 m
  • d: 1 km to 20 km
1 Report ITU-R SM.2028-2 Monte Carlo simulation methodology for the use in sharing and compatibility studies between different radio services or systems, section 6.1, published June 2017.