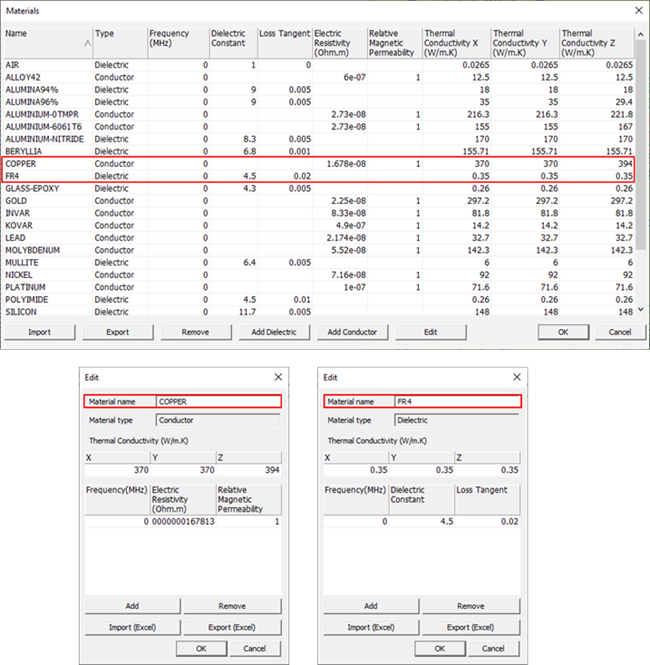
Material Libraries
From the menu bar, click . The Materials dialog opens. Built in and user defined material library can be managed using this menu. Use the Materials dialog to add, modify, and remove certain materials and export the materials data as Material File (*.mtrl) which could be used by import function.
By double-clicking a certain material in the Materials dialog or selecting and then clicking Edit, the material properties can be modified. Clicking Add Dielectric or Add Conductor opens the Edit dialog with empty values.
For conducting material, user should define the electric resistivity, relative magnetic permeability and thermal conductivity along to x, y and z axis. For dielectric material, dielectric constant, loss tangent and thermal conductivity along to x, y and z axis would be needed.
The short descriptions for the representative properties of dielectric and conductive materials are in table below.
| Properties | Description |
|---|---|
| Conductivity | Electrical conductivity or specific conductance is a measure of a
material's ability to conduct an electric current. When an
electrical potential difference is placed across a conductor, its
movable charges flow, giving rise to an electric current. The
conductivity σ is defined as the ratio of the current density
to the electric field strength
: |
| Resistivity | Electrical resistivity ρ (Greek: rho) is defined
by,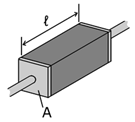 |
| Magnetic Permeability | In electromagnetism, permeability is the measure of the ability
of a material to support the formation of a magnetic field within
itself. In other words, it is the degree of magnetization that a
material obtains in response to an applied magnetic field. Magnetic
permeability is typically represented by the Greek letter
. The reciprocal of magnetic
permeability is magnetic reluctivity. In SI units, permeability is measured in the henry per meter (H m-1), or newton per ampere squared (N A-2). The permeability constant ( , also known as the magnetic constant or the permeability of free space, is a measure of the amount of resistance encountered when forming a magnetic field in a classical vacuum. The magnetic constant has the exact value = 4π×10−7 ≈ 1.2566370614...×10−6 H·m-1 or N·A-2). |
| Thermal Conductivity | Thermal conductivity is the quantity of heat transmitted through a unit thickness in a direction normal to a surface of unit area, due to a unit temperature gradient under steady state conditions. Thermal conductivity is same term with heat transfer coefficients. 1 W/(mK) = 1 W/(moC) = 0.85984 kcal/(hr moC) = 0.5779 Btu/(ft hr oF) |
| Dielectric Constant | A dielectric is an electrical insulator that may be polarized by
the action of an applied electric field. When a dielectric is placed
in an electric field, electric charges do not flow through the
material, as in a conductor, but only slightly shift from their
average equilibrium positions causing dielectric polarization.
Because of dielectric polarization, positive charges are displaced
along the field and negative charges shift in the opposite
direction. This creates an internal electric field which partly
compensates the external field inside the dielectric. If a
dielectric is composed of weakly bonded molecules, those molecules
not only become polarized, but also reorient so that their symmetry
axis aligns to the field. While the term insulator refers to a low degree of electrical conduction, the term "dielectric" is typically used to describe materials with a high polarizability. The latter is expressed by a number called the dielectric constant. A common, yet notable, example of a dielectric is the electrically insulating material between the metallic plates of a capacitor. The polarization of the dielectric by the applied electric field increases the capacitor's capacitance. |
| Loss Tangent | The loss tangent is a parameter of a dielectric material that quantifies its inherent dissipation of electromagnetic energy. The term refers to the angle in a complex plane between the resistive (lossy) component of an electromagnetic field and its reactive (lossless) component. |